Do the property maths properly - and easily

House prices are rising. But be clear about how fast yours is gaining by using our easy and handy calculator

Property

House prices are rising. But be clear about how fast yours is gaining by using our easy and handy calculator

1st Sep 20, 2:03pm by David Chaston

It might be a barbecue conversation, or a dinner table item, but it comes up often - how much has your house value risen?

It's easy to mentally work out the gain in dollar terms. But we all know that this has happened over time.

And if you compare one situation with another, the only clear way to reconcile them is to have a time-adjusted gain - a compound rate per year. Everyone intuitively understands that.

We have a simple calculator that can help.

Enter your starting value, the value now, and the years between the two.

From first principles, you could work it out from this formula:

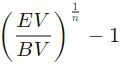
Or you could just use our handy tool, here. Much easier.

The result of either gives an answer smoothed of the volatility in-between the starting value and the ending one, and that is probably what you want to know anyway.

*This calculator was developed by Calculate.co.nz and is in our calculator toolbox as part of our partnership with them.

We welcome your comments below. If you are not already registered, please register to comment

Remember we welcome robust, respectful and insightful debate. We don't welcome abusive or defamatory comments and will de-register those repeatedly making such comments. Our current comment policy is here.

18 Comments

by DoTheMath | 1st Sep 20, 4:22pm 1598934128

I approve this message

by Yvil | 1st Sep 20, 5:37pm 1598938631

Great tool, thanks. Shows nicely the power of compounding, you don't need a high yearly % increase to get very large gains over 10 years and more

by J.C. | 1st Sep 20, 6:21pm 1598941309

Insurance salespeople used to be good with the ol' compounding demonstration.

by Albert2020 | 1st Sep 20, 6:05pm 1598940343

Surely you have to take into account the costs (interest, rates, opportunity cost of deposit) of “ownership” if this was an owner occupied house to produce your net capital gain?

by Noncents | 1st Sep 20, 6:20pm 1598941245

I don't think you can take into account opportunity cost. But it would be fair to include the compulsory costs;
- Interest
- Rates
- House insurance

and I think there could also be an argument to potentially factor in some form of repair/maintenance over the duration of the mortgage.

Do all that, compare to inflation over the same period... and yes, I think it is pretty clear that any form of Capital Gains tax, value tax, etc... on a family home is unfairly punative.

by Lanthanide | 2nd Sep 20, 4:40am 1598978400

But if there were taxes on houses, house prices would be lower to help offset that (since demand for accommodation would be unchanged). Mortgages would be lower, so interest costs would be lower, and insurance costs marginally lower.

by Noncents | 2nd Sep 20, 6:53am 1598986394

lol, that old chestnut - taxes lower prices.

by Shoreman | 2nd Sep 20, 9:42am 1598996532

Lanthanide - Taxing anything never makes anything cheaper !

by Noncents | 1st Sep 20, 6:11pm 1598940698

I was quite surprised by the results.

My place is 3.8% per year over 10 years. I did some additional calcs, and if it continues to increase at that rate, there would be a small net loss (compared to interest paid over the life of a standard 30 year mortgage)

Hardly the tax free gains many here whine about.

by refactornz | 2nd Sep 20, 8:44am 1598993054

Though over that time you would also be receiving rental income (if an investment) or imputed rent (if you live in it).

by Noncents | 2nd Sep 20, 8:58am 1598993898

Imputed rent - probably one of the more idiotic economic theories.

Anything can have an imputed value. So why only houses?

Should we apply the same logic to Cars? Food? Water? Air? Clothing? What about income when I work on my Garden/Car/House? The kids toys? Tools in my shed?

by Te Kooti | 2nd Sep 20, 9:38am 1598996312

If you don't own and occupy, aren't you paying rent (or living in parents basement)?

3.8% may not sound spectacular, but if you had bought the house with a 25% deposit that is an ROE of 15.2%.

by refactornz | 2nd Sep 20, 9:43am 1598996632

I'm not saying that it should be taxed. Just pointing out that there is additional value there.
In this article and comments we're talking about the return on housing, so cars, food, water, air, clothing, etc., are irrelevant in this context.

by Nzdan | 2nd Sep 20, 7:55am 1598990126

17.42% over three years. *shrug*

by David Chaston | 2nd Sep 20, 10:01am 1598997698

Thank you to the reader who contacted us about the headline typo.

by MortgageBelt | 2nd Sep 20, 5:51pm 1599025900

Instead of buying 5 or 10 years ago, could you have instead saved a bigger deposit at the same rate of your house inflation?

by Whatwillhappen | 3rd Sep 20, 9:07am 1599080839

Need an extra step to subtract capital expenditure over the period.

by Epicjuice | 8th Sep 20, 1:12pm 1599527569

Is this nominal or inflation-adjusted?